March 2011

Crossovers: The Short Version
The pros and cons of crossing over. Article By Jeff Poth
Article By Jeff Poth

  Crossovers are the hardest part of a loudspeaker. If you read here, you're likely already aware of the basic idea of a crossover, that being to pass only the desired frequencies to a driver- highs to the tweeter, lows to the woofer, etc. But crossovers also need to perform shaping of the driver responses, compensate for impedance behavior, match relative levels, and other functions. This article should help in interpreting what exactly a crossover does and how. Crossovers are a huge subject, so we'll get you started and give you the tools to begin advanced crossover design, but this is by no means an exhaustive write-up. Hopefully the reader will achieve familiarity with some concepts so that further research will be fruitful; some links are included at the end.

There are three critical components to a crossover, amplitude (SPL), Phase, and impedance (the frequency dependent load presented, similar to "resistance" but with a complex reactive nature). The crossover's task is to create a desired SPL response, but the byproduct of amplitude shaping is phase shifting, and the impedance has to be considered throughout.

Amplitude
Amplitude is how loud a driver will play for a given frequency with a given voltage. This is the first consideration in designing a crossover, we want to get a flat (or some other target) frequency response across the desired bandwidth (range of frequencies). Ideally, you want your woofer, midrange, and tweeter to create a smooth response, and you achieve this by cutting mids and highs out of the woofer, lows and highs out of the midrange, and lows and mids out of the tweeter. The crossover needs to do so in such a manner that those cutoffs don't create peaks or dips in the overall frequency response and everything plays together as though it were a single perfect driver. As you can see below, we have a 3-way 2nd order optimal crossover, creating a flat response in summation (black line). The midrange must be wired with inverted polarity due to the phase behavior of the 2nd order filters.

Phase
Almost as critical as amplitude is phase. Just as crossovers affect the output level of a speaker at a given frequency, they also affect the phase response. Phase response is complex, and represents the behavior of a driver in terms of pushing/pulling given an input signal. When 2 signals at the same frequency are 180 degrees different in phase, one is pushing and one is pulling thus they will tend to cancel each other. Incidentally, this is the amount of phase shift that exists in a textbook 2nd order crossover, or when the wires are reversed on a driver. It's common to see driver wires reversed to show how deep this cancellation notch is, when testing for good phase continuity. The larger the cancellation behavior, the better the phase tracking when hooked up correctly. Phase tracking is critical to making sure that all drivers are working together, and when a woofer plays a bass guitar at 150 Hz, the 300, 450, 600 Hz harmonics in the midrange are pushing / pulling along with the 150 Hz, as they're all part of the same instrument.

Note: This push/pull description is somewhat oversimplified but works for our purposes

Impedance
The crossover has to be designed to work against the complex impedance of the loudspeaker. Impedance is the electrical resistance that's frequency-specific, and varies with frequency both within a single driver and a multi-way loudspeaker system. It's also worth considering what the crossover does to the overall impedance of the speaker system, to make sure you're not making a speaker that will be difficult for amplifiers to drive. The Apogee Scintilla, for example, was a 1 ohm loudspeaker and killed many amplifiers. Even big Krell amps needed modifications to drive these monstrosities correctly. 4 and 8 Ohm loudspeakers are the most common nominal values, but even 4 is too low for some amplifiers. The lower the impedance, the more current is needed to drive the loudspeaker. We'll talk more about this below, but suffice it to say the impedance of the driver and the crossover affects its action in the amplitude and phase. Additionally, the values of crossover components must be adapted to the impedance characteristics of the drivers in the system.

Example via a dose of humble pie for the first order aficionado

Your typical super-budget crossover is comprised of a capacitor on the tweeter and an inductor (coil) on the woofer. This is not uncommon even at the high end of audio, though the success of such a scheme is largely dependent upon the drivers used. You can get away with a cap 'n coil crossover in some instances, but by and large they're not worth using. This type of crossover is often called a "first order" crossover, referring to a 6dB/octave slope of the cutoff. This means that above the cutoff point, the woofer's response will decrease by a factor of 6dB for every octave the frequency increases, and likewise the tweeter will decrease by 6dB per octave below the cutoff for each octave the frequency decreases. The phase is shifted to +/- 90 degrees depending on whether you're talking about a capacitor or a tweeter in series with the driver, and the impedance is increased the more the SPL is reduced (the SPL reduction comes from the voltage and current reductions of the increased impedance of the system). Sometimes these crossovers utilize no inductor on the woofer, relying upon the natural rolloff and voicecoil inductance of the woofer to accomplish the cut of the high frequencies

This is known as one of the only crossovers able to properly reproduce a square wave, which is often used as evidence that this is the preferred crossover. Unfortunately it doesn't often work that way in reality. Those who actually get this type of response from their crossovers have to do a lot of work to get it. Thiel is known for their first order crossovers; however, their crossovers are a "true" first order, which means a lot more effort than a cap and coil. They do a lot of impedance correction and other methods to achieve a real first order behavior.

The reason the cap and coil doesn't work (or almost never does) to achieve a first order behavior is that you're working with real world drivers. Their response isn't flat and neither is their impedance. Consider for a moment the action of an inductor in series with a woofer. We'll first define reactance:

Reactance- frequency dependent resistance of capacitors and inductors, measured in Ohms

Inductive reactance Xl = 2piFL   (F= Frequency   L= Inductance in henries)

Capacitive reactance Xc=1/(2piFC)   (F=Frequency   C=Capacitance in Farads)

Say I wanted to have a rolloff at 2 kHz from my nominally 8 Ohm woofer and tweeter. The math tells us that we'd need a 10uF capacitor on the tweeter and a .64mH to have the crossover create a 6dB downpoint at 2 kHz. These are the values needed to add 8 ohms of series reactance to the nominally 8 ohm drivers at 2 kHz. By doubling the impedance, you halve both voltage (-3dB) and current (another -3dB), hence -6dB. You can see that the inductive reactance is proportional to frequency, so each doubling of frequency will double inductive reactance. Likewise capacitors double their capacitive reactance with each halving of frequency.

Something Is Impeding Our Crossover!
If drivers were simple and perfect, the above cap and coil setup would work great, or at least, would work well within the limitations of a perfect first order crossover. In the real world we have complex behavior to consider. Shown is an impedance plot of a (very well behaved) woofer.

You can see that it's not even close to flat. The nominal calculated values for the crossover are now working against a complex load. The inductor placed in series adds 8 ohms to the impedance at 2 kHz, but the impedance is actually 10 ohms at this frequency, so it's slightly less than a 6dB reduction. Further, the Le (voicecoil inductance) of the woofer creates the same sort of rise the inductor does. This means that when the inductive reactance of the coil doubles, the impedance it's working against has also increased. Between 2 kHz and 4 kHz the coil goes from 8 to 16 Ohms, but the driver is also rising, from 10 to 15 Ohms. So you see a much smaller relative change in output. You get slightly less than 6dB of attenuation at 2 kHz, and slightly more than 6dB at 4 kHz. The idea was to get 6dB at 2 kHz and 12dB at 4 kHz however! And it goes beyond that- you can see that in this (typical) woofer the doubling of inductor reactance with frequency is matched with the Le of the woofer, so you'll never have an increase in dB attenuation above the "slightly over 6 dB" number. Matters only get worse when you go lower than our 2 kHz nominal point- you see that the attenuation takes place earlier than you'd like since the woofer impedance is dropping with decreasing frequency, so the series reactance from the coil also isn't ideal lower.

Now that we've examined the effect of a plain inductor in a crossover, and seen how it is not ideal, we need to figure a solution. Luckily for us, there's something called a Zobel network. This is a capacitor and resistor in series with each other and in parallel with the woofer. The purpose of a Zobel is to flatten the woofer's self-inductance to give the inductor a nearly resistive load to work against, restoring it to a (nearly) optimal 6dB slope. See schematic below

This takes care of the top end rise in the woofer impedance from the voicecoil inductance, but reveals another problem- our 8 Ohm woofer… isn't. It's more like 5 to 6 Ohms (this is typical, the valley above the Fs peak is usually only slightly higher than the DCR of a driver). So we need to now adjust our inductor value, as we only need about 5.5 ohms of inductive reactance to achieve a 6dB reduction in level. Accordingly, we would adjust the value down to about .4mH and we have a correctly implemented first order rolloff in the woofer IF the woofer is extremely flat and extended in terms of frequency response. The driver whose impedance is shown is fairly flat and smooth to about 5 kHz (JBL 2213), but your typical 12" will have major frequency response variations much lower in frequency. Even 8"s usually don't cleanly reach that high. That said, so long as the rolloff of the woofer is fairly clean you can usually use a "first" order crossover per the above and achieve good results. 

When examining an impedance curve we still see one other obtrusive feature- the impedance spike at Fs. This is the resonance of the driver in question. Not a major issue for the woofer since you're not trying to apply a high-pass function, so it's not within the crossover operating band. However for a tweeter, or a midrange, this is a significant problem. Most are tuned to keep this impedance rise fairly low, like in the Dayton ND20FB-4 (note the frequency scale, the Fs is at 2 kHz) 

This Fs spike is probably not severe enough to warrant much correction. Note that it's only a 30% rise or so above the impedance minimum (though it takes place quite quickly). Some drivers, however, are much more difficult to work with. JBL 2426h on a horn is one of these. Horn speakers in general tend to have more challenging characteristics to work with and this is no exception.

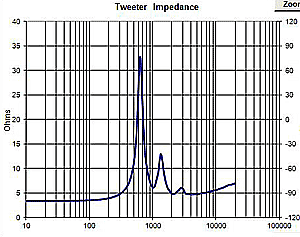
As you can see, there are three individual impedance spikes, two of which are fairly severe. A "textbook" first order crossover would never work with these, and thus the impedance needs significant correction. The correction for a spike, as opposed to a gradual rise like voicecoil inductance, requires a notch filter. This is what's called a tuned circuit as the reactance of the capacitor and inductor effectively enhance each other. For impedance correction it's typical to see an inductor, a capacitor, and a resistor in series with each other, and in parallel with the speaker load. Values are calculated to match the frequencies and Q of the impedance spikes. The effect will be greatest (peak or dip depending on arrangement) at the frequency where the reactance of the capacitor and the inductor are equal. Damping resistors are used in most notch filters to ensure that the spike or dip is of appropriate size. Here you see a pair of notch filters tuned to the impedance spikes of the 2426h. 

As you can see, the peaks are dramatically suppressed, and we now have a relatively stable impedance to work against. You can refine your notches ad infinitum but this was as far as I took this process for this example. Again here, you can see a reduction in nominal load along with the compensation of the impedance, so the capacitor value would need to be increased.

Combine the dramatic shifts in impedance with the nonlinear frequency response of real world loudspeaker drivers, and we see that a cap 'n coil filter is largely useless for high performance systems. It can be used in some cases, but only with a lot of care and refinement, and even then it has some significant downsides vs. more robust crossovers. First order crossovers have a lot of overlapping bandwidth, leading to lobing issues/comb filtering, and increased power handling/excursion demands on the drivers. On the upside, there is minimal phase shift, and the parts quality can be maintained at a very high level. The losses in the crossover can be kept to a minimum, which can be significant in budget sensitive systems where high ESR inductors are used. 

Higher Order Crossovers
We have focused mostly on the shortcomings of the first order crossover thus far. As we get more complex, we add components, filter slope, and more attention to phase requirements. A crossover can be first, second, third, or whatever order ad infinitum. Fourth is about the practical limit for most real-world loudspeakers. Note that you will hear two variants on the slope, electric and acoustic. An electric 4th order crossover is four components per driver and is designed to achieve a 24dB/Octave cutoff. Since most speaker drivers are designed (or incidentally limited) to have a cutoff at the end of their desirable operational bandwidth, a proper crossover design incorporates that cutoff. That cutoff is usually 12dB/Oct. or thereabouts for the first octave of the stopband (where you desire to cutoff the response). What this means is that you can have a 4th order acoustic cutoff with a 2nd order electrical filter, or even sometimes a simple cap or coil (with impedance correction, per above). This is a speaker-by-speaker process, and no hard rules apply, there's far too much variability to pin this down any tighter. Important to note is that the more steep a filter becomes, the more phase shift it has.

High order is nice in that they offer steeper slopes. This means that fewer highs get to the woofer to excite its breakup modes, and less lows come to the tweeter, which is often the reason a tweeter fries. Tweeters need a reasonably steep crossover to prevent both electrical energy and displacement requirements. Small assemblies such as a tweeter cannot dissipate much heat or move very much without damage and steep crossovers minimize both the amount of electrical energy and excursion required. Woofers/Mids often have significant "breakup" modes, frequencies at the high end of their response where the driver suddenly has a jump in output and distortion. This is because of the mechanical resonances in the diaphragm / assembly. Metal cones are the worst in this respect, and damped paper generally is pretty benign. High order filters help ensure that this is effectively suppressed and doesn't show up as harshness. Notch filters can also be used, but in my opinion are overused to suppress breakup. High Q breakups like those of a metal cone can be very hard to damp during dynamic operation.

This Is Hard!
I warned you! Fortunately, you're a lucky person as you have tools available to you that dramatically simplify crossover design (the process, not the schematic!). A combination of measurement and simulation can get you a fairly well-refined loudspeaker without investing a terrible amount of time. I use a Dayton WT3 www.enjoythemusic.com/diy/1108/dayton_wt3.htm for quick impedance measurements. This allows you to assemble a prototype crossover design then quickly measure it to see if you're getting the simulated results. What simulated results? Why, Jeff Bagby's PCD results of course! There are other crossover packages, but Jeff's worksheets have the advantages of being free, powerful, easy, and well-supported. He offers other software as well as seen here . Jeff gave his permission to use his software here (and it was used to generate many of these graphs), and suggested I promote the use of his response modeling software in addition to the crossover designer.

Another Resource
Please also refer to Wayne Parham's (of Pi Speakers) excellent write-up of crossover design (as seen here) used with his permission here. Not surprisingly there's some overlap, but I think you'll find my article to be a very useful sister document to his more technical/example driven paper. Thanks Wayne!

Links
A few more links to get you going. This is not a shallow subject, so keep reading, simulating, then read and simulate some more, and build a few. The top link is a forum post, but don't let that dissuade you. It's from Wayne Parham (as above) and includes a video on testing vertical nulls, very useful for waveguide/horn loudspeaker testing in particular.

www.audioroundtable.com/forum/index.php?t=msg&th=12067

www.linkwitzlab.com/crossovers.htm

www.musicanddesign.com/Duelund_and_Beyond.html

www.musicanddesign.com/Power.html